• About Me
  • Projects
  • Resume
  • Contact
  • More
    • About Me
    • Projects
    • Resume
    • Contact
  • About Me
  • Projects
  • Resume
  • Contact

LUCAS HABER

LUCAS HABERLUCAS HABERLUCAS HABER

Abaqus System Analysis

Utilizing Abaqus, I designed and analyzed a 3D model of a skateboard undergoing high stress applications. The skateboard was designed and tested with an all wood composition and again with a carbon fiber sheet in the central layer to compare stress and strain distribution.

Introduction/Purpose:

Reason For Analysis

I will model a skateboard using the estimated forces of a person landing on it after a 4ft drop. I have chosen this project because I enjoy skateboarding in my free time and have seen people break their skateboards after ollieing off stairs and ledges. 

Skateboard Design

 Skateboards have existed for decades and in that time the design has stayed the same at its core; layers of wood veneer are glued and pressed together to form the board. This design allows for a cheap and easy to produce product that tends to be reliable, even withstanding the forces of some extreme drops. The shear forces from landing with a lot of weight on the board creates cracks throughout the wood grain until it reaches a breaking point. Layering different grains on top of each other helps to mitigate the issue but can’t entirely prevent it. 

Design Alteration

As has been done by incorporating steel beams into concrete slabs, the combination of different materials can also have very beneficial effects. One great example of this is skis. What used to be made exclusively out of wood is now made of many combinations of wood, fiberglass, carbon fiber, aluminum, Kevlar, and more. These materials not only give the skis increased strength, but also different feelings and reactions.


I would like to explore how incorporating some of these same materials into skateboard designs will impact the overall strength and deflection of the board. 

Methods:

Setup

Force Analysis

Force Analysis

  There will be two skateboard models, both will be made of two 0.175in thick wooden sections sandwiching a third, 0.05in layer. In one model, this layer will be made of carbon fiber and in the other it will be made of wood. The layers will be assigned a frictionless global contact property because they are assumed to be glued together.


To reduce analysis time and calculation errors, the model was simplified into 3 flat rectangular layers measuring 8.5x32in.

Force Analysis

Force Analysis

Force Analysis

 I model the skateboard analysis given the assumption that a 170lb person lands on it after a 4ft drop. Assuming an initial velocity of 0 in/s, their velocity by the time they reach the ground will be 192.5 in/s. Assuming the person crouches down 6in as they land, thus increasing their collision distance, the average impact force will be 1360lbf over an assumed 0.001 seconds. This force will be distributed evenly at two points, one at the front-center of the board and another at the board’s tail. These assumptions are made to simulate and simplify the expected forces that would act on the board when landing after an ollie. 

Material Choice

Modeled Assumptions

Modeled Assumptions

For the wooden layers of the skateboard I’m using maple wood, the most popular material for constructing skateboards. Maple has a Young’s Modulus of 1.83e+6 psi and Poisson’s Ratio of 0.424 for deformation along the radial axes due to stress along the longitudinal axis. The density is 0.0224 lb/in^3. As it is flexible and durable, it can easily be formed to the necessary shape for the board and has a long lifecycle before chipping or cracking.


For the reinforced core of the skateboard, I have chosen to use carbon fiber. Standard carbon fiber has a Young’s Modulus of 1.015e+7 psi and a Poisson’s Ratio of 0.10. The density is 0.0723 lb/in^3.  It is up to 5 times stronger than steel and has nearly twice the stiffness with a fraction of the weight, making it the perfect material to reinforce a skateboard. 

Modeled Assumptions

Modeled Assumptions

Modeled Assumptions

  There will be two assumed static sections on the skateboard where the trucks normally meet the board. These sections will be modeled as static because the ground will be stopping the trucks in place, thus holding the board stationary at those points at the time of impact.


The impact will be modeled as a Dynamic, Explicit step with a time period of 0.001 seconds. This should resemble the forces that would act on the skateboard during the initial impact with the ground when the forces should be the highest.

Results:

  By plotting the seed value against the displacement, I was able to come up with a trendline for their relationship, giving me the equation f(x) to use in my convergence analysis. My analysis, shown below, gave me a seed value of ~2.45 at convergence, producing 42 elements per skateboard layer.

Wooden Skateboard

Reinforced Skateboard

Reinforced Skateboard

In the fully wooden skateboard, the maximum Von Mises stress of 4944psi occurs at the base of the front trucks where the system is fixed in place. Using the modulus of rupture for maple wood, 15800psi, this point has a factor of safety of 3.196. 

Reinforced Skateboard

Reinforced Skateboard

Reinforced Skateboard

In the skateboard with the reinforced carbon fiber core, the maximum Von Mises stress of 17840psi occurs within the core at the point where the system is fixed in place. Using the yield strength of carbon fiber, 362590psi, this point has a factor of safety of 20.324.

Discussion:

Stress Analysis

Stress Analysis

Stress Analysis

  

Figure 1: Wood Core Layer. Maximum Stress – Wooden Core

Stress Analysis

Stress Analysis

  

Figure 2: Carbon Layer, Maximum Stress - Carbon Core

The carbon layer absorbs much of the stress of impact, giving it a higher stress than any of the layers in the wood-core board. This is not an issue though because the strength of the carbon is far greater than that of the wood, greatly reducing the chance of failure.


Additionally, after analyzing the displacement of the skateboard, the carbon core would significantly reduce the flex of the board as seen in figures 4 and 5 below.

Strain Analysis

Strain Analysis

Strain Analysis

  

  

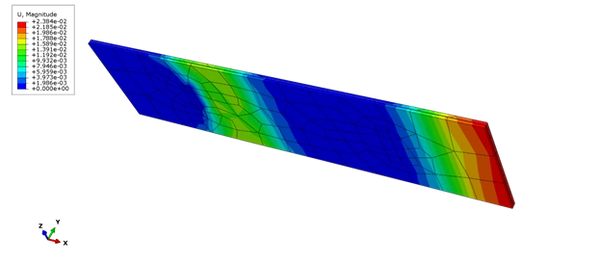
Figure 3: Wooden Core, Displacement Magnitude

Strain Analysis

Strain Analysis

  

  

Figure 4: Carbon Core, Displacement Magnitude

  The maximum displacement when using the carbon core is 78.52% of the displacement using the maple wood core. This reduction in the flexing of the board reduces the bending forces that act on the wooden layers of the board, reducing the overall chance of snapping.


The modeling of the mechanism is fairly accurate because the fixed points for the trucks are given rotational freedom. This is since in real life these points are free to rotate slightly as the board flexes with the wheel as the center of rotation. One limitation of this model is that the fixed points are just points and not a fixed surface.


This was done because if the entire surface was fixed then the freedom of rotation would have been lost. Additionally, in real life some of the impact force would have been absorbed by the rubber of the wheels and some of the joints in the skateboard trucks. Since this could not have been modeled without a much more complex system, these factors were not considered. The analysis is also limited by the simplification of the model’s shape. This was done, as stated above, to allow the analysis to properly function without error or extreme processing time. 

Conclusion:

As shown in figure 3, the carbon core of the skateboard would absorb much of the force of impact. As analyzed in the results section, it would increase the factor of safety of the core from 3.196 to 20.324. Figures 4 and 5 further show that overall flex in the skateboard would be reduced, lowering the probability of any fractures in the wood due to bending.


In terms of marketability, carbon fiber skateboards can cost anywhere between $100-300. This makes them 2-6 times as expensive as a traditional wooden skateboard making it a less appealing price point. Additionally, some skaters may be apprehensive of the stiffness of the skateboard. Many skaters appreciate the flex and give that a traditional skateboard has which makes it easier on the joints. A stiff skateboard will have a completely different feel for the rider and will translate more of the impact forces into the rider’s body. While the increase in the factor of safety is significant, many skaters may be unwilling to pay the higher price and may not enjoy the stiffer feeling of the board. 

Copyright © 2024 Lucas Haber - All Rights Reserved.

Powered by GoDaddy Website Builder